
Escher + Alhambra = Infinity
- Arts
- Architecture
11
Written by Ana M. Carreño Leyva
Art courtesy of The M.C. Escher Company
“I can’t say how my interest in the regular division of planes originated and whether outside influences had a primary effect on me. My first intuitive step in that direction had already been taken as a student … before I got to know the Moorish majolica mosaics in the Alhambra, which made a profound impression on me.”
—M.C. Escher, 1941
One evening just more than 100 years ago—October 19, 1922, to be exact—a 24-year-old artist from the Netherlands named Maurits Cornelius Escher sat down in the 14th-century meeting hall of the Alhambra palace in Granada, Spain. There he began to sketch the patterns of the tiles that filled its walls. He later wrote in his travel diary that he was so spellbound he spent the entire evening there copying an intricate, 16-point star mosaic, and that he did not stop until he had completed it with watercolors the next day. “It was amazingly oriental,” he wrote. “What strikes me is the remarkable richness of its decoration (stucco low relief) and the great dignity and simple beauty throughout the place … What is strange in this decoration is the complete absence of any human or animal shape, even of any vegetal form; this is maybe what gives it strength.” Later he reflected, “I became more and more intrigued by the fitting together of congruent figures.”

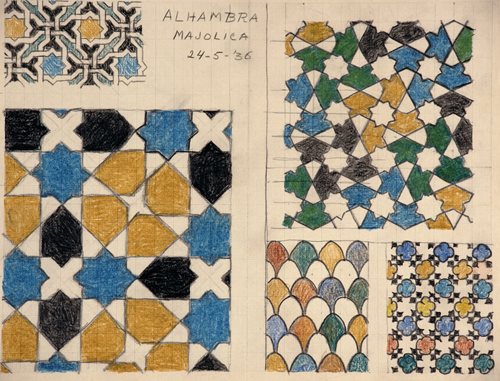
Fourteen years later, this was to be the reason of his return to Granada for three days in May 1936, this time together with his wife, Giulietta, or Jetta. He and Jetta both filled pages with notes and sketches. He marveled at how “the Moors were masters in the art of filling a plane with similar, interlocking figures.” The sketches from this trip inspired increasingly complex experiments with repetitive patterns of human- or animal-shaped figures that he later extended into three dimensions through use of hyperbolic (curved) planes.
Rafael Pérez-Gómez, a professor of mathematics at the University of Granada and an expert on Escher, points out that despite Escher’s lack of formal training in mathematics or any field of science, he remains the artist most admired by mathematicians. He explains that Escher “found in the Granadan monument the same universe he intended to create for himself though the endless combination of geometrical forms.”
Escher’s interest was entirely practical, Pérez-Gómez explains. He was fascinated by how the artisans of the Alhambra had found solutions to representation that avoided empty spaces without using figurative images while, at the same time, “conveying the unity of divine presence in the universe, as well as its endlessness.” Nonetheless, tiling and tessellation—the filling of a space with an infinitely repeatable pattern—was a subject of less interest to Escher at the time than the still lifes, townscapes, book designs and illustrations that were helping him earn a living to support Jetta and the couple’s two sons. He was not interested in classifications or categorizations; rather, he wanted to get to know what governed the patterns in the Alhambra so he could create his own ways to apply regular divisions to a graphic plane.



Escher began his experiments with repeatable patterns as early as 1920, center, and he produced his print “Eight Heads” left shortly before his trip to Spain in 1922. Right “Procession in a Crypt,” 1927: The interior space of infinite naves marked by columns and vaults evoke both Christian churches and Muslim mosques and mark Escher’s interest in 3D patterns, which later led to complex constructions of endless stairs, Möbius strips and impossible figures.
This fascination with the regular division of a plane remained like a plant that was inadvertently growing, one he would not fully recognize and cultivate until the latter 1930s. Then it became his leading source of inspiration, and he nurtured it by practicing, drawing, experimenting and experiencing—a process of pure persistence he carried on for decades.
As he played with combining and twisting parallelogram grids and interlocking polygons shaped as humans, lizards, fish, birds, frogs, monkeys and more, he also began to develop landscapes that integrated such figures into perspectives that were impossible from a rational, physical point of view. He also had taken note of how the Arab artisans and mathematicians of the Alhambra had used color to enhance the symmetry of their designs: “It has always been self-evident for the Moors to compose their tile scenes with pieces of majolica in contrasting colors. Likewise, I have never hesitated myself to use color contrast as a means of visually separating my adjacent pattern components,” wrote Escher in 1941.


Escher did not come to his passions in isolation. Born in 1898, he was imbued by the experience of sciences within his family from childhood. In one of his letters, Escher admitted, “my father and three of my brothers were all trained in the exact sciences or engineering, and I have always had an enormous respect for these things.” Most notably it was his brother Berend, a professor of geology and later rector of the University of Leiden in the Netherlands, who in the later 1930s introduced him to crystallography, the field that studies the structures of crystalized minerals.
But Escher himself struggled as a young student. “I had, and still have,” he wrote in 1941, “great trouble with abstractions of numbers and letters. Things went a little better in geometry when I was called upon to use my imagination, but I never excelled in this subject either while at school.”
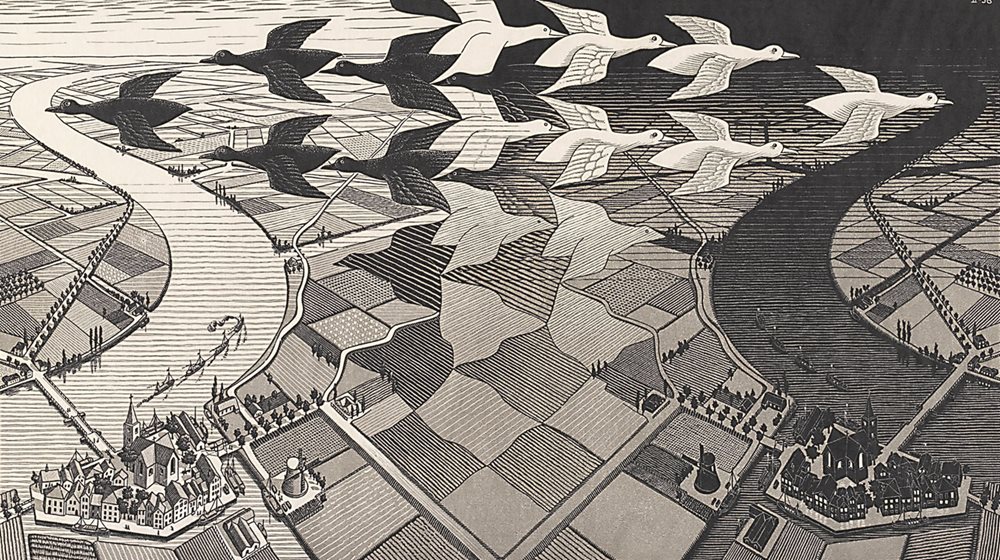


Right Pérez-Gómez points to the pattern Escher used as a foundation for “Angels and Demons” left, and one of his most famous lithographs, “Day and Night” top, produced in 1938. “The ‘discovery’ of such a pattern that, according to a certain system, repeats itself rhythmically and as such depends on unbreakable laws, has always been and continues to bring me great joy,” Escher wrote in 1936. “It offers the sensation of approaching something both ancient and eternal.”
Escher was 21 when, in September 1919, he enrolled at the Haarlem School for Architecture and Decorative Arts. Encouraged there by the noted Dutch graphic artist Samuel Jessurun de Mesquita, Escher began making woodcuts in which dual images interlocked by means of quarter- and half-rotations. In 1922, months prior to his trip to Spain, he made a black-and-white woodcut he titled “Eight Heads” that prefigured much about his later work. It was his first flat newspaper design.
Escher enhanced his self-training by reading and studying scientific articles on the regular division of the plane, too. In crystallography he was inspired by the figures developed by Hungarian mathematician George Pólya, “Über die Analogie der Kristallsymmetrie in der Ebene” (“On the Analogy of Crystallographic Symmetry in the Plane”), which categorized 17 flat periodic mosaics whose symmetries corresponded to the 17 Planar Crystallographic Groups. It was then that Escher began associating these natural patterns with those he had seen not only in the Alhambra, but also in the mosque of Córdoba, the Reales Alcázar in Seville, as well as at sites in Italy.


Pérez Gómez points out that all 17 of these crystallographic patterns appear in the designs at the Alhambra. “The hidden keys to decipher the Moorish designs of the Alhambra are basically found on the following bases, established on three points,” he says. “First, the existence of a basic lattice that extends throughout the plane by the action of a crystallographic group; second, the basic grid can be designed in infinite ways, keeping only the area invariant; third, the size of the basic grid is related to the number of colors in the tile.”
“I often have wondered at this, for an artist, unusual mania of mine to design periodic drawings. Over the years I made about 150 of them … I was simply driven by the irresistible pleasure I felt in repeating the same figures on a piece of paper. I had not yet seen the tile decorations of the Alhambra, and never heard of crystallography; so I did not even know that my game was based on rules that have been scientifically investigated.”
As a prolific engraver, Escher produced a massive number of lithographs, woodcuts and myriads of drawings and sketches. His journey through the dynamic geometry of the plane continued, Pérez-Gómez says, “until he made the regular division of the hyperbolic plane using the Euclidean model given by the French mathematician Henri Poincaré. Escher saw an image that caught his attention in an article by Canadian mathematician Harold Scott Coxeter, “Crystal Symmetry and its Generalizations,” and Escher wrote to him asking about its geometric construction. In 1954 Escher met Coxeter in Amsterdam at the International Congress of Mathematicians, and the artist and the mathematician maintained a friendship until Escher’s death in 1972. Coxeter was instrumental to Escher’s understanding of how a hyperbolic-plane geometric model can be constructed: The result was Escher’s Circle Limit series. For example, “Circle Limit IV” is equivalent to the earlier, flat-plane pattern “Angels and Devils,” but made on the model of the hyperbolic plane with right triangles with angles of 30 degrees, 45 degrees and 90 degrees. With these angles, these triangles are only possible in hyperbolic geometry since their angles add up to more than 180 degrees. “It is more than surprising to think that its origin is in the tiled mosaic of the Alhambra,” as is one of Escher’s best-known images, “Day and Night,” says Pérez-Gómez.

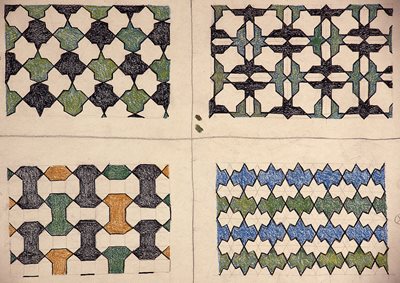
What makes Escher’s “extra-logical realities” so compelling? Is it the search of equilibrium amid the duality of infinity versus limited space, enhanced through black and white dichotomies? Is it symmetry by means of rhythmic repetitions and a combination of colors that made figurative shapes appear kinetic and vibrant? Both were strategies to the contrary yet complementary along with the potential for infinity just outside our perception of the physical world. He kept them in balance by using the same proportions of colors, so as not to have one steal prominence but for each to boost the others. By playing with space and time, he suggests the ways reality may surpass our senses, how by a trompe l’oeil of geometry our perception of reality can be manipulated. In his own words, he assured his viewers that he was not interested in “reality” and that his aim was not to express any feeling nor transmit any message; his medium was not in the humanities, but in the abstract language of mathematics used to pose paradoxes and offer up spatial mysteries.
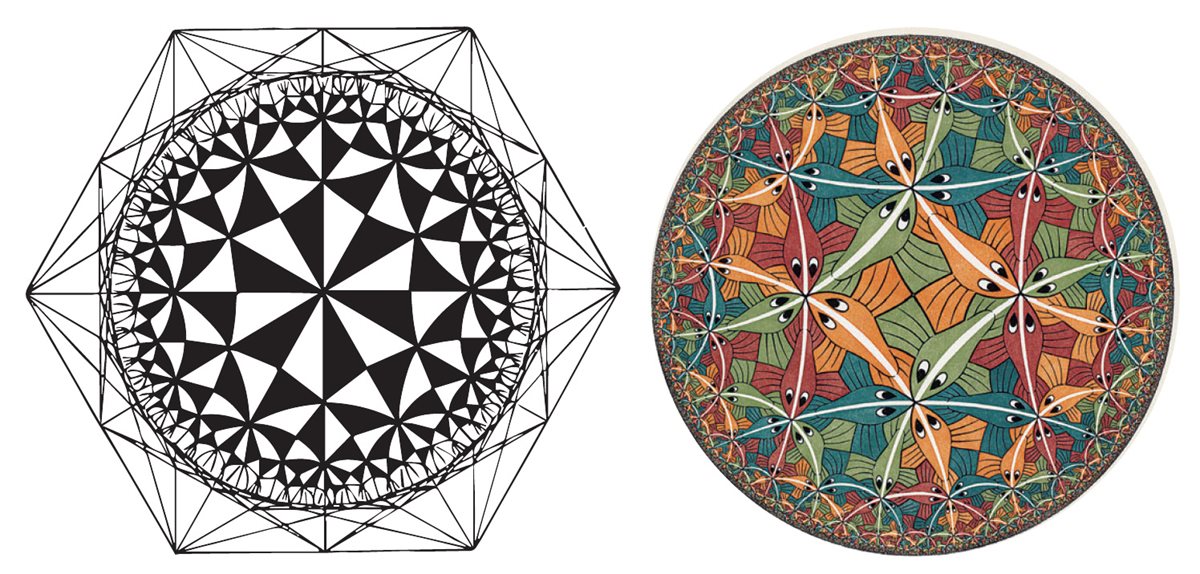
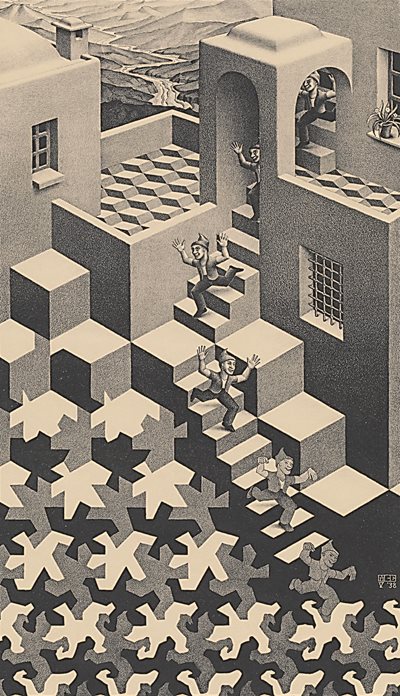
Escher’s work has been an object of academic study that has generated more than a dozen lines of investigation in mathematics as the so-called “Escher algorithm”: patterns that arise from triangles, quadrilaterals and hexagons whose shapes change while they maintain their areas in ways similar to the periodic mosaics in the Alhambra.
From his early interests in patterns to his systematic study of the Alhambra, the mosque at Córdoba, he took crystallographic geometry toward spatial infinity and later, through hyperbolic planes, pushed practical geometry into the fourth dimension of time. This put his works well within the framework of 20th century European vanguardist art movements as Dadaism, Surrealism and Op Art, with the result that his drawings have been endlessly reproduced in popular culture, from vinyl LP covers to wall posters and fabric designs.
Escher’s works invite our eyes to travel never-ending landscapes and to walk spaces with no beginnings and no ends, where horizons fold into foregrounds. We are left awestruck by seemingly impossible complexity, born deep within the imagination of an “artist who painted the infinite,” says Pérez-Gómez—one whose inspiration was forever stimulated by patterns designed six centuries before his lifetime on the walls of the Alhambra.
You may also be interested in...

The Bridge of Meanings
History
Arts
There is no truer symbol of Mostar, Bosnia-Herzegovina, than its Old Bridge. The magnificent icon of Balkan Islamic architecture was destroyed during the 1992–’95 war—but not for long. Like the multicultural workforce that produced the original hundreds of years earlier, a broad team of architects, engineers and others came together immediately to plan its reconstruction. This summer marked the 20th anniversary of the bridge’s reopening.
Meet Me at the Mudhif
History
Arts
Dozens of volunteers joined together in Houston, Texas, to construct a mudhif, a reed structure dating back 5,000 years to the Mesopotamian marshes of southern Iraq. To this day the hut serves as a town hall for Marsh Arabs to meet with their sheikh. In Houston, it also served as a meeting place--for some of the city's 4,000 Iraqis and their fellow Houstonians to share insights into an ancient society and gain a sense of community.
2022 Calendar: Muqarnas
Arts
Dazzling, prismatic and intricately symmetrical, muqarnas is a stunning three-dimensional decoration unique to Islamic architecture.